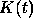 be given by Eq. (3), and let
be given by Eq. (3), and let


Let 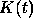 be given by Eq. (3), and let
be given by Eq. (3), and let
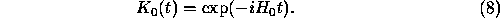
Then 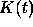 satisfies the Schrödinger equation
satisfies the Schrödinger equation
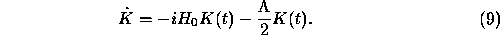
This differential equation, together with initial data 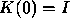 , is easily seen to be
equivalent to the following integral equation:
, is easily seen to be
equivalent to the following integral equation:
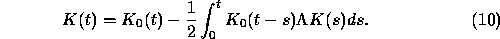
By taking the Laplace transform and by the convolution theorem we get the Laplace transformed equation:
Let us consider the case of a maximally sharp measurement. In this case
we would take 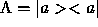 , where |a> is some Hilbert space vector.
It is not assumed
to be normalized; in fact its norm stands for the strength of the coupling
(notice that <a|a> must have physical dimension
, where |a> is some Hilbert space vector.
It is not assumed
to be normalized; in fact its norm stands for the strength of the coupling
(notice that <a|a> must have physical dimension 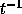 ).
Taking look at the formula (4)
we see that now
).
Taking look at the formula (4)
we see that now  and so we need to know
and so we need to know
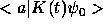 rather than the full propagator
rather than the full propagator 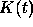 . Multiplying Eq. (11)
from the left by <a| and from the right by
. Multiplying Eq. (11)
from the left by <a| and from the right by 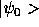 we obtain:
we obtain:
where 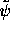 is the Laplace transform of
is the Laplace transform of 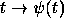 :
: